Skip to main content

Postmodern Algebra

Sam Macdonald

Section 6.4 Projectives and Injectives

Subsection Projectives, Injective, and Resolutions: Revisited

A lot of the notions we have studied this semester can be extended to the setting of a general abelian category.

Definition 6.69.

Definition 7.49. Let \(\mathcal{A}\) be an abelian category. An object \(P\) in \(\mathcal{A}\) is projective if \(\operatorname{Hom}_{\mathcal{A}}(P,-)\) is an exact functor. An object \(E\) in \(\mathcal{A}\) is injective if \(\operatorname{Hom}_{\mathcal{A}}(-, E)\) is exact.
This generalizes the notion of projective and injective modules.

Remark 6.70.

Remark 7.50. Let \(\mathcal{A}\) be an abelian category. An object \(P\) is projective if and only if every arrow \(P \longrightarrow Y\) factors through every epi \(X \longrightarrow Y\) :
and an object \(E\) is injective if and only if every arrow \(X \longrightarrow E\) factors through every mono \(X \longrightarrow Y\) :

Exercise 6.71.

Exercise 93. Let \(\mathcal{A}\) be an abelian category.
a) Show that \(\operatorname{Hom}_{\mathcal{A}}(x \oplus y, z)=\operatorname{Hom}_{\mathcal{A}}(x, z) \oplus \operatorname{Hom}_{\mathcal{A}}(y, z)\text{.}\)
b) Show that if \(P\) and \(Q\) are projective, then so is \(P \oplus Q\text{.}\)

Definition 6.72.

Definition 7.51. An abelian category \(\mathcal{A}\) has enough projectives if for every object \(M\) there exists a projective object \(P\) and an epi \(P \longrightarrow M\text{.}\) We say that \(\mathcal{A}\) has enough injectives if for every object \(M\) there exists an injective object \(E\) and a mono \(M \longrightarrow E\text{.}\)
Lemma 4.13 and Theorem 4.31 say that \(R\)-Mod has enough injectives and enough projectives.

Example 6.73.

Example 7.52. The category of finite abelian groups has no projectives beside 0. In particular, Ab does not have enough projectives.

Definition 6.74. Projective Resolution.

Definition 7.53. Let \(M\) be an object in the abelian category \(\mathcal{A}\text{.}\) A projective resolution of \(M\) is a complex
\begin{equation*} \cdots \longrightarrow P_{2} \longrightarrow P_{1} \longrightarrow P_{0} \longrightarrow 0 \end{equation*}
where all the \(P_{n}\) are projective, \(\mathrm{H}_{0}(P)=M\text{,}\) and \(\mathrm{H}_{n}(P)=0\) for all \(n \neq 0\text{.}\) An injective resolution of \(M\) is a cochain complex
\begin{equation*} 0 \longrightarrow E^{0} \longrightarrow E^{1} \longrightarrow \cdots \end{equation*}
such that every \(E^{n}\) is injective, \(\mathrm{H}^{n}(E)=0\) for all \(n\text{,}\) and \(\mathrm{H}^{0}(E)=M\text{.}\)

Proof.

Proof. Given be an object \(M\) in \(\mathcal{A}\text{,}\) let’s construct a projective resolution explicitly. We start by picking an epi \(P_{0} \stackrel{\varepsilon}{\rightarrow} M\) from a projective \(P_{0}\text{.}\) Since \(\epsilon\) is an epi, it is the cokernel of its kernel, so
\begin{equation*} 0 \longrightarrow \operatorname{ker} \varepsilon \longrightarrow P_{0} \stackrel{\varepsilon}{\longrightarrow} M \longrightarrow 0 \end{equation*}
is a short exact sequence. Now we find an epi \(P_{1} \stackrel{\varepsilon_{1}}{\longrightarrow} K_{0}:=\operatorname{ker} \varepsilon\text{,}\) and set \(P_{1} \xrightarrow{\partial_{1}} P_{0}\) to be the composition
We proceed the same way, at each step taking a projective \(P_{n}\) and an epi \(\varepsilon_{n}: P_{n} \longrightarrow \operatorname{ker} \partial_{n-1}\text{,}\) and setting \(\partial_{n+1}\) to be the composition \(\left(\operatorname{ker} \partial_{n-1}\right) \circ \varepsilon_{n}\text{.}\) By construction, \(\partial_{n}=i_{n-1} \varepsilon_{n}\text{,}\) where \(\varepsilon_{n}\) is an epi and ker \(\partial_{n-1}\) is mono. By Exercise 85, im \(\partial_{n}=i_{n-1}=\operatorname{ker} \partial_{n-1}\text{.}\)
This generalizes Theorem 5.2 in a natural way, and the proof is essentially the same.

Subsection Split Exact Sequences: Revisited

We can also characterize injectives in term of split short exact sequences, as we did for modules. In particular, the Splitting Lemma extends to any abelian category.

Definition 6.76.

Definition 7.55. Let \(\mathcal{A}\) be an abelian category. A short exact sequence
\begin{equation*} 0 \longrightarrow A \xrightarrow{f} B \xrightarrow{g} C \longrightarrow 0 \end{equation*}
splits if one of the following equivalent conditions hold:
  1. There exists an arrow \(C \stackrel{r}{\rightarrow} B\) such that \(g r=\operatorname{id}_{C}\text{.}\)
  2. There exists an arrow \(C \stackrel{r}{\rightarrow} B\) such that \(g r=\mathrm{id}_{C}\text{.}\)
  3. There exists an isomorphism of complexes between our sequence and
\begin{equation*} 0 \longrightarrow A \longrightarrow A \oplus C \longrightarrow C \longrightarrow 0 \end{equation*}
where the arrows are the canonical arrows that come with the (co)product \(A \oplus C\text{.}\)
The proofs are exactly the same as in the case of \(R\)-Mod, Theorem 4.6 and Theorem 4.32. Proof. If \(C\) is projective, there exists \(h\) such that
commutes, so \(g h=\operatorname{id}_{C}\) and \(g\) is a splitting. If \(A\) is injective, there exists \(h\) such that
commutes, so \(h f=\operatorname{id}_{A}\text{,}\) and \(h\) is a splitting.
More generally, we can talk about split exact complexes.

Definition 6.78.

Definition 7.57. A complex \(C\) in \(\operatorname{Ch}(\mathcal{A})\) is split if there are arrows \(s_{n}: C_{n} \longrightarrow C_{n+1}\) such that the differential \(\partial\) satisfies \(\partial=\partial s \partial\text{.}\) A complex is split exact if it is both exact and split.

Remark 6.79.

Remark 7.58. A split short exact sequence is precisely a short exact sequence that is a split complex.

Exercise 6.80.

Exercise 94. Additive functors preserve split complexes, meaning that if \(C\) is a split complex, then so is \(F(C)\) for any additive functor \(F\text{.}\) In particular, additive functors preserve split short exact sequences.

Subsection Unique Resolutions and the Horseshoe Lemma: Revisited

Proof.

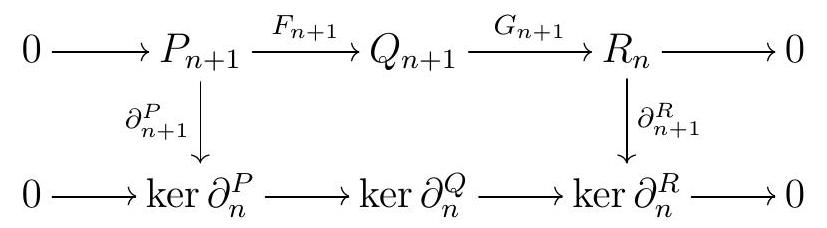
Proof. Since \(P_{0}\) is projective and \(\delta_{0}\) is an epi, there exists \(\varphi_{0}\) such that
commutes.
We proceed inductively, assuming we have \(\varphi_{0}, \ldots, \varphi_{n-1}\) with \(\varphi_{n-2} \partial_{n-1}=\delta_{n-2} \varphi_{n-1}\text{.}\) Since \(P_{n}\) is projective, there exists \(\varphi_{n}\) such that
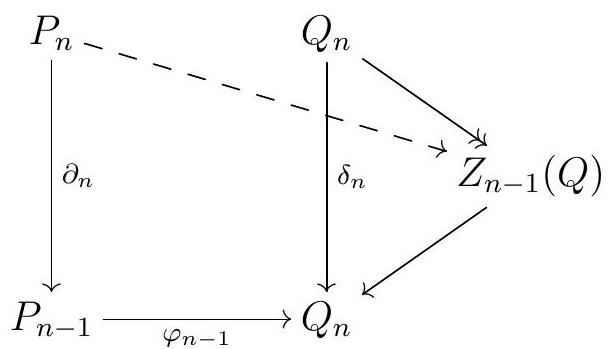
commutes. Commutativity gives \(\delta_{n-1} \varphi_{n-1} \partial_{n}=\varphi_{n-2} \partial_{n-1} \partial_{n}=0\text{,}\) so \(\varphi_{n-1} \partial_{n}\) factors through the kernel of \(\delta_{n-1}\text{.}\)
Since \(Q\) is a projective resolution of \(N\text{,}\) the arrow \(Q_{n} \longrightarrow Z_{n-1}(Q)\) above is an epi, so the arrow \(P_{n} \longrightarrow Z_{n-1}(Q)\) we just constructed factors through \(Q_{n}\text{,}\) giving us \(\varphi_{n}\) such that
commutes.
Now suppose we are given two such maps of complexes \(\varphi\) and \(\psi\) lifting \(f\text{,}\) say \(\varphi\) and \(\psi\text{.}\) Note that \(\varphi-\psi\) and 0 are two liftings of the 0 map. We are going to show that any map lifting the 0 map \(M \longrightarrow N\) must be nullhomotopic, which will then imply that \(\varphi\) and \(\psi\) are homotopic as well (essentially via the same homotopy!).
So let \(\varphi: P \longrightarrow C\) be a map of complexes lifting the 0 map \(M \longrightarrow N\text{.}\)
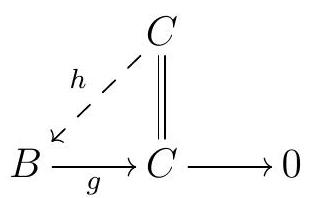
We will construct a nullhomotopy for \(\varphi\) inductively. Set \(h_{n}=0\) for all \(n<0\text{.}\) The commutativity of the rightmost square says that \(\delta_{0} \varphi_{0}=0\text{,}\) so \(\operatorname{im} \varphi_{0} \subseteq \operatorname{ker} \delta_{0}=\operatorname{im} \delta_{1}\text{.}\) Since \(\partial_{0} \varpi_{0}=0, \varphi_{0}\) factors through \(Z_{0}(Q)\text{.}\) But \(Q_{1} \rightarrow Z_{0}(Q)\) is an epi and \(P_{0}\) is projective, there exists \(H_{0}\) such that
commutes. So \(H_{0}\) satisfies \(\delta_{0} H_{0}=\varphi_{0}\text{.}\) Set \(H_{-1}=0\text{.}\)
Now suppose we have constructed \(H_{0}, \ldots, H_{n-1}\) such that \(\delta_{n} H_{n-1}+H_{n-2} \partial_{n-1}=\varphi_{n-1}\text{.}\) Then
\begin{equation*} \begin{aligned} \delta_{n} \varphi_{n} & =\varphi_{n-1} \partial_{n} & \text { since } \varphi \text { is a map of complexes } \\ & =\left(\delta_{n} H_{n-1}+H_{n-2} \partial_{n-1}\right) \partial_{n} & \text { by assumption } \\ & =\delta_{n} H_{n-1} \partial_{n}+H_{n-2} \partial_{n-1} \partial_{n} & \\ & =\delta_{n} H_{n-1} \partial_{n} & \text { since } \partial_{n-1} \partial_{n}=0 \end{aligned} \end{equation*}
so \(\delta_{n}\left(\varphi_{n}-H_{n-1} \partial_{n}\right)=0\text{.}\) Therefore, \(\varphi_{n}\) factors through \(Z_{n}(Q)\text{,}\) and since \(Q\) is a projective resolution of \(N, Q_{n+1} \longrightarrow Z_{n}(Q)\) is an epi. Therefore, the factorization of \(\varphi_{n}-H_{n-1} \partial_{n}\) through \(Z_{n}(Q)\) also factors through \(Q_{n}\text{,}\) and we end up with an arrow \(H_{n}\) such that
commutes. This \(H_{n}\) must then satisfy \(\delta_{n-1} H_{n}+H_{n-1} \partial_{n}=\varphi_{n}\text{,}\) and ultimately \(H\) is a homotopy between \(\varphi\) and 0 .

Proof.

First, a word on notation: \(\oplus\) denotes the coproduct in \(\mathcal{A}\text{,}\) and given arrows \(x \stackrel{f}{\rightarrow} z\) and \(y \stackrel{g}{\rightarrow} z\text{,}\) we will write \(f \oplus g\) for the unique arrow \(x \oplus y \longrightarrow z\) induced by \(f\) and \(g\text{.}\) Moreover, we will denote the differential of \(P\) by \(\partial^{P}\text{,}\) and the differential of \(R\) by \(\partial^{R}\text{.}\)
Set \(Q_{n}=P_{n} \oplus R_{n}\text{.}\) Recall that the product and coproduct in \(\mathcal{A}\) coincide, by Lemma 7.10, so let \(F_{n}: P_{n} \longrightarrow Q_{n}\) and \(G_{n}: Q_{n} \longrightarrow R_{n}\) be the canonical arrows. One can show that in fact we get short exact sequences
\begin{equation*} 0 \longrightarrow P_{n} \stackrel{F_{n}}{\longrightarrow} Q_{n} \stackrel{G_{n}}{\longrightarrow} R_{n} \longrightarrow 0 \end{equation*}
for all \(n\text{.}\) Moreover, \(Q_{n}\) is projective for all \(n\text{,}\) by Exercise 93. We will construct the missing differentials \(\partial^{Q}\) inductively.
Since \(R_{0}\) is projective and \(g\) is an epi, there exists \(\gamma\) such that
commutes. Set \(\partial_{0}^{Q}:=\left(f \partial_{0}^{P}\right) \oplus \gamma\text{.}\) The universal property of the coproduct guarantees that
commutes. By the Five Lemma, \(\partial_{0}^{Q}\) is epi. By the Snake Lemma,
\begin{equation*} \operatorname{ker} \partial_{0}^{P} \longrightarrow \operatorname{ker} \partial_{0}^{Q} \longrightarrow \operatorname{ker} \partial_{0}^{R} \end{equation*}
is exact. We then proceed by induction, and at each step we apply the base case to
where the vertical arrows are epi because \(P\) and \(R\) are projective resolutions and thus exact.

Remark 6.83.

By duality, if \(\mathcal{A}\) has enough injectives,
\begin{equation*} 0 \longrightarrow A \longrightarrow B \longrightarrow C \longrightarrow 0 \end{equation*}
is exact, and \(E_{A}\) and \(E_{C}\) are injective resolutions for \(A\) and \(C\text{,}\) then there exist an injective resolution \(E_{B}\) of \(B\) and a short exact sequence of complexes
\begin{equation*} 0 \longrightarrow E_{A} \longrightarrow E_{B} \longrightarrow E_{C} \longrightarrow 0 \end{equation*}
extending the given one.
PrevTopNext